| Issue |
Ann. For. Sci.
Volume 67, Number 4, June 2010
|
|
|---|---|---|
| Article Number | 409 | |
| Number of page(s) | 6 | |
| DOI | https://doi.org/10.1051/forest/2009129 | |
| Published online | 02 April 2010 | |
Original article
Elastic parameters of poplar wood with end-cracks
Les constantes élastiques du bois de peuplier fissuré aux extrémités
1
Agriculture Research Center (KIAU-ARC) Islamic Azad
University, Karaj Branch Moazen
BLVD, Rajaee-shahr, P.O. Box
31485
Karaj, Iran
2
Department of Wood Science & Technology, Islamic Azad
University, Karaj Branch Moazen
BLVD, Rajaee-shahr,
P.O. Box 31485
Karaj, Iran
* Corresponding author:
mehran.roohnia@kiau.ac.ir
Received:
16
March
2009
Accepted:
5
September
2009
• In this study, longitudinal specific modulus of elasticity along the grain (specific MOEL = MOEL/ρ) as well as radial and tangential shear moduli (GLR and GLT) of Populus Deltoides wood were examined in free flexural vibration in a free-free bar method, where end-cracks produced manually in LT plane along and parallel to annual rings in four different sizes.
• The effects of four different crack sizes (0, 6, 12, and 18 cm) on elastic parameters of the bars were examined for their vibration properties based on Timoshenko bar equations, in order to find a procedure to make a confident choice of a clear specimen among the cracked ones, considering three initial modes of vibration.
• Based on research findings, a significant correlation existed between radial and tangential shear moduli of the clear bars as GLR was approximately 15 percents higher than GLT. After making the shortest crack sizes, however, this correlation entirely faded. Statistically for 6 cm crack, decreases in specific MOEL for measurement on the tangential impact were not significant though they were for longer cracks.
• It was also revealed that in specimen under the study if longitudinal specific modulus of elasticity from both LR and LT flexural vibrations were almost equal and GLR was slightly larger than GLT, the user could be confident enough to consider the specimen without severe longitudinal cracks.
Résumé
• Dans cette étude, le module d’élasticité longitudinal spécifique (MOEL/ρ) et les modules de cisaillement radial et tangentiel (GLR and GLT) du bois de Populus deltoides ont été mesuré en vibration de flexion libre-libre pour quatre différentes longueurs de fissures initiées manuellement dans le plan LT qui est parallèle au fil du bois et tangentiel aux limites de cernes.
• Les effets des quatre différentes tailles de fissures (0, 6, 12 et 18 cm) sur les constantes élastiques des barres ont été examinées pour leurs propriétés vibratoires sur la base des équations de Timoshenko, dans le but de trouver une procédure conduisant à un choix fiable d’un échantillon sans défauts parmi les échantillons fissurés, ceci en considérant les trois modes initiaux de vibration.
• Les résultats obtenus montrent une corrélation significative entre les modules de cisaillement radial et tangentiel des barres sans défauts, GLR étant environ 15 pour cent plus élevé que GLT. Cependant après avoir réalisé les fissures les plus courtes cette corrélation n’existe plus. Pour une fissure de 6 cm, la décroissance du module longitudinal spécifique mesuré en tangentiel n’est pas significative alors que c’était le cas pour des fissures plus longues.
• Pour nos échantillons, nous avons également montré que si les modules d’élasticité longitudinaux spécifiques sont presque identiques et GLR légèrement plus important que GLT, l’utilisateur peut exploiter en confiance les échantillons sans fissures importantes.
Key words: crack / damage / elasticity / free vibration / Populus Deltoides
Mots clés : fissure / endommagement / élasticité / vibration libre / Populus deltoides
© INRA, EDP Sciences, 2010
1. INTRODUCTION
After knots, cracks are the most important defects in wood and wood composites which cause limitations in their performance. Consequently, the effects of cracks on wood performance have been the prime interest in most mechanical testing. In addition to standard tests, non-destructive methods have recently been used to evaluate the mechanical parameters and their behaviors.
In this field, vibration-based techniques are widely known as fast, reliable, and inexpensive procedures for crack identification (Loutridis et al., 2005). Since a crack affects the dynamic behavior of a bar by reducing the natural frequencies and the probable mechanical derivatives, examining these changes makes it possible to understand the effects of cracks size and position on mechanical properties.
Recently, the effects of cracks shape, position and behavior during the vibration have widely been investigated. Similarly, the instantaneous frequency and empirical mode of decomposition for crack detection in cantilever beams with breathing cracks have been studied (Loutridis et al., 2005). In another research, the free and forced vibrations for crack detection in cantilever beams were examined and it was proved whereas free vibration analysis could provide valuable information to single and multiple cracks detection, forced vibration could only detect the single cracks (Orhan, 2007). However, it was argued that dynamic response in forced vibration could better describe the changes in crack depth and location comparing to free vibration in which the differences between natural frequencies corresponding to a change in crack depth and location only indicated minor effects. Along the same line, the vibrations caused by two impact shocks and the signals in both defect-free and cracked bars were analyzed and compared in a frequency domain (Cam et al., 2005). The research findings offered a procedure for determining the depth and location of cracks by analyzing the vibration signals. Moreover, the experimental results out of dynamic behavior of a circular arch in several undamaged and damaged configurations have been reported and they were compared with those obtained by means of analytical methods; acceptable agreement was observed between the analytical and experimental results and an identification procedure based on frequency measurements was proposed and validated (Cerri et al., 2008). Modal-based damage detection algorithm for identifying the location and extent of the defects in timbers was the subject of a recent study that came up with some modifications to the existing defect detection algorithms. In this study, researchers used the two initial modes of vibration to compare the modal strain energy before and after damage (Choi et al., 2007). In order to compare the mode shapes of vibration before and after damage in timbers, modal-based testing was applied on wood bars in order to propose a new statistical algorithm for obtaining a defect indicator (Hu et al., 2006). Last but not least, free vibration testing was used to generate the two initial mode shapes for damage detection in timbers and it was proved that the chosen Daubechies 3 wavelet was suitable and sufficiently sensitive to identify the location, extent and number of different damages (Hu et al., 2006).
As far as research in the field is concerned, longitudinal cracks have seldom been noticed. To examine other types of imperfections, the evaluation of longitudinal cracks in wood bars in terms of modulus of elasticity and the shear modulus was foreseen in the current study. Experiments on theoretical predictions were carried out in order to testify the significant effects of the longitudinal cracks on decreasing the modulus of elasticity and the shear modulus in poplar wood or presence of any positive relationship between them.
2. MATERIALS AND METHODS
The specific longitudinal modulus of elasticity
(MOEL/ρ) and the shear modulus can be
evaluated based on Timoshenko bar equations and Bordonné Solution (Bordonné, 1989; Brancheriau et al., 2002). In this equation, after obtaining the kth frequency
through Fourier Transform, considering ak and
bk, the value of longitudinal specific
modulus of elasticity was determined using a linear regression formula as following:
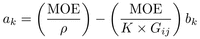 (1)
(1)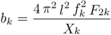 (2)
(2)![\begin{equation} \mathop a\nolimits_k =\frac{[4\mathop \pi \nolimits^2 \mathop l\nolimits^2 \mathop f\nolimits_k^2 (1+\alpha \mathop F\nolimits_{1k} )]}{\mathop {\alpha X}\nolimits_k } \label{eq3} \end{equation}](/articles/forest/full_html/2010/04/f09110/f09110-eq3.gif) (3)
(3) (4)
(4) (5)where I; moment of inertia,
A; cross section area, l; length of the specimen,
K; shape coefficient (the value of 5/6 can be used for a rectangular
cross section), Gij; shear modulus in plane of
vibration (GLT or
GLR), ρ; specific gravity,
fk; frequency of the kth
mode of vibration obtained from FFT spectrum
mk; the kth results in
following equation:
(5)where I; moment of inertia,
A; cross section area, l; length of the specimen,
K; shape coefficient (the value of 5/6 can be used for a rectangular
cross section), Gij; shear modulus in plane of
vibration (GLT or
GLR), ρ; specific gravity,
fk; frequency of the kth
mode of vibration obtained from FFT spectrum
mk; the kth results in
following equation: 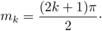 (6)In Equations (1) and (2),
F1k and
F2k can be calculated as following:
(6)In Equations (1) and (2),
F1k and
F2k can be calculated as following:
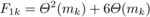 (7)
(7)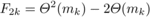 (8)
(8)![\begin{equation} %eq9 \Theta _{(m_k )} =\frac{[m_k \tan (m_k)\tanh (m_k)]}{[\tan (m_k )-\tanh (m_k )]}\cdot \end{equation}](/articles/forest/full_html/2010/04/f09110/f09110-eq9.gif) (9)Higher correlation coefficients of the estimated trend
lines in Equation (1) benefit the specimens with more homogeneity, where the Timoshenko
model has been fitted initially to isotropic materials, and next to the clearest specimens.
The selection was made based on trends with correlation coefficients higher than 0.99.
(9)Higher correlation coefficients of the estimated trend
lines in Equation (1) benefit the specimens with more homogeneity, where the Timoshenko
model has been fitted initially to isotropic materials, and next to the clearest specimens.
The selection was made based on trends with correlation coefficients higher than 0.99.
In this study, Eastern poplar (Populus Deltoides) timbers were randomly collected from plantations in Zanjan province in the region between West Alborz and North Zagros Mountains. Eastern poplar wood is a common commercial wood with widespread use in Iran. Following ISO 3129 international standard (1975), 25 rectangular and visually clear wooden bars (specimens) were obtained. The specimens were cut to their final nominal dimensions of 2 × 2 × 36 cm, R × T × L, and kept in a conditioning chamber at 21 °C and 65 percents relative humidity for two weeks until their moisture content was stabilized. In controlled pathways, the 0, 6, 12 and 18 cm cracks parallel to annual rings were produced with a carpentry chisel in longitudinal directions. The cracks were visible on opposite radial surfaces (Fig. 1).
 |
Figure
1 Cracks in plane with LT surface of a 2 × 2 × 36 cm R × T × L bar. Crack production, controlled in a proper pathway. Look to procedure A to D. |
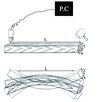 |
Figure 2 Schematic view of the most common setups for free flexural vibration on a free-free bar test. Sound recording from cracked end and hammer impact on other free end of a bar leaned on two soft thin supports, 0.22L from each end. |
Free flexural vibration on free-free bars, rested on soft thin rubber, was performed for all crack sizes. Individual impacts on either radial or tangential surface excited the bar to vibrate in LT and LR planes respectively (Fig. 2). The impacts were applied on the whole end where the microphone was located on the cracked end over the center of both crack legs. Vibrations were recorded as audio files at sampling rate of 44100 Hz. Every selected and saved acquisition sound file contained 14000 to 18000 points, depending on the attenuation through the time that was less than a second. After reading the audio files at the same sampling rates, the three initial modes of vibration were obtained from magnitude of Fourier Transform spectrum in MATLAB v.7.1 (Fig. 3).
To analyze the obtained data, some statistical tests were conducted in SPSS 11.5. Using one-way analysis of variances (ANOVA) followed by Duncan multiple comparison tests, the effects of step-wise crack propagation on elastic parameters were analyzed and the presence of significant correlations in clear bars before damage was studied during and after the crack production from one free end into the middle of the bars.
 |
Figure 3 Three initial modes of vibration-magnitude of a Fourier Transform. Y axis corresponding to amplitude in dB and X axis the frequency in Hz. |
3. RESULTS AND DISCUSSION
Prior to presenting the research findings, a discussion on the definition of elastic modulus seems necessary. Elastic modulus is usually defined as a material property but in this study it is specifically defined as the response of a bar. In the former case, the property itself does not change after geometrical manipulations of the bar, although the response of the bar would be affected. However, when the bar is under impact on its transverse surface, the vibration is excited in LR plane. The bar legs frequently hit each other so that it eventually results in fading the resonance frequency. Therefore, decreasing the natural frequencies would decrease the elastic parameters due to their positive correlations with the resonance frequencies in Timoshenko bar equations.
 |
Figure 4 Comparison of longitudinal specific modulus of elasticity (MPa/Kg/m3), results of vibrations in LR and LT planes of clear bars (A) statistically, the constant in linear equation was not significant; of 6 cm cracked bars (B); of 12 cm cracked bars (C); of 18cm cracked bars(D). Based on 18 observations. |
In the current study, since only one longitudinal specific modulus of elasticity was adopted for a normal grain wood, there would be almost equal estimates out of vibrations in LT or LR planes before the presence of any defects. However in some cases, small differences could be observed since probably some differences existed in MOE between the early and late woods. This would lead to a higher MOE out of vibration in LR plane rather than vibration in LT plane. Apart from these small and dispensable differences, after producing cracks at least one of the vibrations would result in smaller natural frequencies; hence smaller values of the longitudinal specific modulus of elasticity (Loutridis et al., 2005). It is worth mentioning that non-destructive vibration method is suitable in case of isotropic materials or in orthotropic directions. A defect which could fade homogeneities would result in weakening the present correlations.
In the next step, longitudinal specific modulus of elasticity as well as two shear moduli of 25 samples for individual crack sizes was measured. Based on Timoshenko bar equation, closely considering correlation coefficients higher than 0.99, 18 out of 25 measurements were accepted as the clearest ones and taken into account for further analyses. The effects of different crack sizes on each elastic parameter were examined using one-way analysis of variances (ANOVA) followed by Duncan multiple comparison tests (Supplementary Tables S1–S3 available at www.afsjournal.org). Even though cracks occasionally caused the vibration test unsuccessful in elastic parameters evaluation (mostly due to low correlation coefficients in linear regression equations and some unexpected negative values) when the specimen was vibrated in LT plane (parallel to crack plane) it was possible to predict the crack sizes had no significant effects on elastic parameters. This could be due to the free vibration of each crack leg so that the homogeneity barely changed in LT plane. Theoretically, the elastic parameters were not expected to be affected by cross-sectional dimensions.
Statistical analysis showed that smaller cracks (e.g., 6 cm or even 12 cm cracks) did not significantly decrease the specific modulus of elasticity (Sup. Table S2). Meanwhile, the Pearson product moment correlations between the longitudinal specific modulus and the results of vibrations in LR against LT plane proved the stable equality of the two obtained series even at the 6cm cracked bars.
In larger crack sizes, the correlation completely lost its significance (Fig. 4). The results, therefore, indicated that changes in specific modulus of elasticity cannot be relied on for identifying the smaller cracks.
In the first step of crack production, a decrease in GLR was initially observed (Tab. S3). In Figure 5, the correlation coefficients for two series of shear moduli are shown where GLR was approximately 15 percents higher than GLT in the non-cracked clear bars. As the length of the crack increased, however, this correlation was entirely lost (Fig. 5). The results also showed that in a proper direction, the numerical finger prints of initial longitudinal cracks on shear modulus might be applicable in crack identification, because even in non-cracked clear specimens there was not a significant correlation between GLT and the longitudinal modulus of elasticity (MOEL). Opposite to GLT, GLR showed highly significant correlation. Upon starting the first step of LT crack production, however, the described correlation for GLR entirely faded (Fig.6).
 |
Figure 5 Comparison of shear moduli, GLR and GLT, in clear bars, the non-significant constant in linear equation (A); in 6 cm cracked bars (B). Based on 18 observations. |
 |
Figure 6 Correlations between radial shear modulus (GLR) and longitudinal modulus of elasticity (MOEL). Results of vibration in LR plane in clear bars (A). Significant constant in linear equation, in 6 cm cracked bars (B). Based on 18 observations. |
This study, therefore, has introduced a new approach to the phenomenon that would happen if the vibration was excited in the cracked end and measured in the whole end of the bar. This new approach, however, was discovered right after the ending point of crack production when the smaller cracks were not available anymore. The authors barely noticed any important differences between two opposite ends for the microphone and excitation place but to them it seems critical to re-examine the research findings in early future.
4. CONCLUSION
This study was carried out to investigate the accuracy of predictions for the positive effects of crack length on elastic moduli of poplar wood when the vibration was perpendicular to the crack surface. This assumption was experimentally proved to be true, though the desirable effects were exclusively traced in longer cracks. The research findings indicated that the changes in the modulus of elasticity and its specific values were not statistically reliable finger prints for identifying the smaller cracks in the poplar wood.
As it was expected, the initial crack size in this study affected the shear modulus in perpendicular direction to the crack plane (i.e., LT crack and GLR. Therefore it can be concluded that finger prints of initial longitudinal cracks on shear modulus in proper directions might be applicable for crack identification. In case the longitudinal modulus of elasticity obtained in both vibrations in LT and LR planes would not be nominally equal to one another, only in individual specimen of poplar wood under study, there would be enough reasons for defects (i.e., crack) existence.
Finally, if both of the above-mentioned longitudinal moduli of elasticity were nominally equal to one another and the GLR was nominally slightly larger than the GLT, the user could be confident enough that his specimen is without severe longitudinal cracks.
Online material
Appendixes
ANOVA for the effects of the step-wise (0 to 18 cm) crack propagation on elastic parameters.
Duncan multiple comparison tests for the Longitudinal Specific MOE (MPa/Kg/m3) obtained from vibration in LR plane at different crack length-Based on 18 observations.
Duncan multiple comparison tests for the GLR obtained from vibration in LR plane at different crack lenghth (GPa)–Based on 18 observations.
Acknowledgments
Our special thanks go to Dr. Iris Bremaud and Dr. Loic Brancheriau, expert scientists from Montpellier, France, for their kind and valuable comments during the course of experiments, Dr. Ahmad Jahan-latibari and Dr. Natasha Qale from Islamic Azad University, Karaj Branch for their assistance with the English revisions, and finally we sincerely thank Mr. Rahi Gaffarian from Islamic Azad University, Karaj Branch and Ms. Negin Manouchehri from University of Tehran for their caricatures and drawings.
References
- Bordonné P.A., 1989. Module dynamique et frottement intérieur dans le bois: Mesures sur poutres flottantes en vibrations naturelles. Ph.D. thesis, INP Lorraine, Nancy, 154 p. [Google Scholar]
- Brancheriau L., and Bailleres H., 2002. Natural vibration analysis of clear wooden beams: a theoretical review, Springer-Verlag. Wood Sci. Technol. 36: 347–365. [CrossRef] [Google Scholar]
- Cam E., Orhan S., and Luy M., 2005. An analysis of cracked beam structure using impact echo method. NDT E Int. 38: 368–373. [Google Scholar]
- Cerri M., Dilena N., and Ruta G.C., 2008. Vibration and damage detection in undamaged and cracked circular arches: experimental and analytical results. J. Sound Vibr. 314: 83–94. [CrossRef] [Google Scholar]
- Choi F.C., Li J., Samali B., and Crews K., 2007. Application of modal-based damage-detection method to locate and evaluate damage in timber beams. J. Wood Sci. 53: 394–400. [CrossRef] [Google Scholar]
- Hu C., and Afzal M.T., 2006. A statistical algorithm for comparing mode shapes of vibration testing before and after damage in timbers. J. Wood Sci. 52: 348–352. [CrossRef] [Google Scholar]
- Hu C., and Afzal M.T., 2006. A wavelet analysis-based approach for damage localization in wood beams. J. Wood Sci. 52: 456–460. [CrossRef] [Google Scholar]
- Loutridis S., Douka E., and Hadjileontiadis L.J., 2005. Forced vibration behaviour and crack detection of cracked beams using instantaneous frequency. NDT E Int. 38: 411–419. [Google Scholar]
- Orhan S., 2007. Analysis of free and forced vibration of a cracked cantilever beam. NDT E Int. 40: 443–450. [Google Scholar]
- Wood – Sampling Methods and General Requirements for Physical and Mechanical Tests – 1975 – 11 – 01 – International Standard ISO 3129. [Google Scholar]
All Tables
ANOVA for the effects of the step-wise (0 to 18 cm) crack propagation on elastic parameters.
Duncan multiple comparison tests for the Longitudinal Specific MOE (MPa/Kg/m3) obtained from vibration in LR plane at different crack length-Based on 18 observations.
Duncan multiple comparison tests for the GLR obtained from vibration in LR plane at different crack lenghth (GPa)–Based on 18 observations.
All Figures
 |
Figure
1 Cracks in plane with LT surface of a 2 × 2 × 36 cm R × T × L bar. Crack production, controlled in a proper pathway. Look to procedure A to D. |
| In the text | |
 |
Figure 2 Schematic view of the most common setups for free flexural vibration on a free-free bar test. Sound recording from cracked end and hammer impact on other free end of a bar leaned on two soft thin supports, 0.22L from each end. |
| In the text | |
 |
Figure 3 Three initial modes of vibration-magnitude of a Fourier Transform. Y axis corresponding to amplitude in dB and X axis the frequency in Hz. |
| In the text | |
 |
Figure 4 Comparison of longitudinal specific modulus of elasticity (MPa/Kg/m3), results of vibrations in LR and LT planes of clear bars (A) statistically, the constant in linear equation was not significant; of 6 cm cracked bars (B); of 12 cm cracked bars (C); of 18cm cracked bars(D). Based on 18 observations. |
| In the text | |
 |
Figure 5 Comparison of shear moduli, GLR and GLT, in clear bars, the non-significant constant in linear equation (A); in 6 cm cracked bars (B). Based on 18 observations. |
| In the text | |
 |
Figure 6 Correlations between radial shear modulus (GLR) and longitudinal modulus of elasticity (MOEL). Results of vibration in LR plane in clear bars (A). Significant constant in linear equation, in 6 cm cracked bars (B). Based on 18 observations. |
| In the text | |


